गणित में त्रिकोणमितीय फलनों के प्रतिलोम फलनों को प्रतिलोम त्रिकोणमितीय फलन (inverse trigonometric functions) कहते हैं। इनके डोमेन समुचित रूप से सीमित करके पारिभाषित किये गये हैं। इन्हें sin−1 , cos−1 आदि के रूप में निरूपित करते हैं और 'साइन इन्वर्स', 'कॉस इन्वर्स' आदि बोलते हैं।
arcsin x = y {\displaystyle \operatorname {arcsin} \ x=y} sin y = x {\displaystyle \operatorname {sin} \ y=x} arccos x = y {\displaystyle \operatorname {arccos} \ x=y} cos y = x {\displaystyle \operatorname {cos} \ y=x} arctg x = y {\displaystyle \operatorname {arctg} \ x=y} tg y = x {\displaystyle \operatorname {tg} \ y=x} arcctg x = y {\displaystyle \operatorname {arcctg} \ x=y} ctg y = x {\displaystyle \operatorname {ctg} \ y=x} arcsec x = y {\displaystyle \operatorname {arcsec} \ x=y} sec y = x {\displaystyle \operatorname {sec} \ y=x} arccsc x = y {\displaystyle \operatorname {arccsc} \ x=y} csc y = x {\displaystyle \operatorname {csc} \ y=x} उदाहरण:
arcsin 0 = 0 {\displaystyle \operatorname {arcsin} \ 0=0} arcsin 0.5 = π 6 {\displaystyle \operatorname {arcsin} \ 0.5={\frac {\pi }{6}}} arcsin 1 = π 2 {\displaystyle \operatorname {arcsin} \ 1={\frac {\pi }{2}}} arccos 0 = π 2 {\displaystyle \operatorname {arccos} \ 0={\frac {\pi }{2}}} arccos 0.5 = π 3 {\displaystyle \operatorname {arccos} \ 0.5={\frac {\pi }{3}}} arccos ( − 1 ) = π {\displaystyle \operatorname {arccos} (-1)=\pi } arctg 0 = 0 {\displaystyle \operatorname {arctg} \ 0=0} arctg 1 = π 4 {\displaystyle \operatorname {arctg} \ 1={\frac {\pi }{4}}} arcctg 0 = π 2 {\displaystyle \operatorname {arcctg} \ 0={\frac {\pi }{2}}} arcctg 1 = π 4 {\displaystyle \operatorname {arcctg} \ 1={\frac {\pi }{4}}}
चूँकि कोई भी त्रिकोणमितीय फलन एकैकी (one-to-one) नहीं है, इनके प्रतिलोम फलन तभी सम्भव होंगे यदि इनके डोमेन सीमित रखे जांय।
निम्नांकित सारणी में मुख्य प्रतिलोमों का विवरण दिया गया है-
नाम सामान्य निरूपण परिभाषा वास्तविक परिणाम के लिये x का डोमेन मुख्य मानों का परास (रेंज) रेडियन ) मुख्य मानों का परास arcsine y = arcsin x x = sin y −1 ≤ x ≤ 1 −π/2 ≤ y ≤ π/2 −90° ≤ y ≤ 90° arccosine y = arccos x x = cos y −1 ≤ x ≤ 1 0 ≤ y ≤ π 0° ≤ y ≤ 180° arctangent y = arctan x x = tan y all real numbers −π/2 < y < π/2 −90° < y < 90° arccotangent y = arccot x x = cot y all real numbers 0 < y < π 0° < y < 180° arcsecant y = arcsec x x = sec y x ≤ −1 or 1 ≤ x 0 ≤ y < π/2 or π/2 < y ≤ π 0° ≤ y < 90° or 90° < y ≤ 180° arccosecant y = arccsc x x = csc y x ≤ −1 or 1 ≤ x −π/2 ≤ y < 0 or 0 < y ≤ π/2 -90° ≤ y < 0° or 0° < y ≤ 90°
यदि x को समिश्र संख्या होने की छूट हो तो y का रेंज केवल इसके वास्तविक भाग (real part) पर ही लागू होगा।
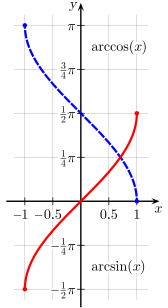
The usual principal values of the arcsin(x ) (red) and arccos(x ) (blue) functions graphed on the cartesian plane. The usual principal values of the arctan(x ) and arccot(x ) functions graphed on the cartesian plane. Principal values of the arcsec(x ) and arccsc(x ) functions graphed on the cartesian plane. Complementary angles:
arccos x = π 2 − arcsin x {\displaystyle \arccos x={\frac {\pi }{2}}-\arcsin x} arccot x = π 2 − arctan x {\displaystyle \operatorname {arccot} x={\frac {\pi }{2}}-\arctan x} arccsc x = π 2 − arcsec x {\displaystyle \operatorname {arccsc} x={\frac {\pi }{2}}-\operatorname {arcsec} x} Negative arguments:
arcsin ( − x ) = − arcsin x {\displaystyle \arcsin(-x)=-\arcsin x\!} arccos ( − x ) = π − arccos x {\displaystyle \arccos(-x)=\pi -\arccos x\!} arctan ( − x ) = − arctan x {\displaystyle \arctan(-x)=-\arctan x\!} arccot ( − x ) = π − arccot x {\displaystyle \operatorname {arccot}(-x)=\pi -\operatorname {arccot} x\!} arcsec ( − x ) = π − arcsec x {\displaystyle \operatorname {arcsec}(-x)=\pi -\operatorname {arcsec} x\!} arccsc ( − x ) = − arccsc x {\displaystyle \operatorname {arccsc}(-x)=-\operatorname {arccsc} x\!} Reciprocal arguments:
arccos ( 1 / x ) = arcsec x {\displaystyle \arccos(1/x)\,=\operatorname {arcsec} x\,} arcsin ( 1 / x ) = arccsc x {\displaystyle \arcsin(1/x)\,=\operatorname {arccsc} x\,} arctan ( 1 / x ) = 1 2 π − arctan x = arccot x , if x > 0 {\displaystyle \arctan(1/x)={\tfrac {1}{2}}\pi -\arctan x=\operatorname {arccot} x,{\text{ if }}x>0\,} arctan ( 1 / x ) = − 1 2 π − arctan x = − π + arccot x , if x < 0 {\displaystyle \arctan(1/x)=-{\tfrac {1}{2}}\pi -\arctan x=-\pi +\operatorname {arccot} x,{\text{ if }}x<0\,} arccot ( 1 / x ) = 1 2 π − arccot x = arctan x , if x > 0 {\displaystyle \operatorname {arccot}(1/x)={\tfrac {1}{2}}\pi -\operatorname {arccot} x=\arctan x,{\text{ if }}x>0\,} arccot ( 1 / x ) = 3 2 π − arccot x = π + arctan x , if x < 0 {\displaystyle \operatorname {arccot}(1/x)={\tfrac {3}{2}}\pi -\operatorname {arccot} x=\pi +\arctan x,{\text{ if }}x<0\,} arcsec ( 1 / x ) = arccos x {\displaystyle \operatorname {arcsec}(1/x)=\arccos x\,} arccsc ( 1 / x ) = arcsin x {\displaystyle \operatorname {arccsc}(1/x)=\arcsin x\,} If you only have a fragment of a sine table:
arccos x = arcsin 1 − x 2 , if 0 ≤ x ≤ 1 {\displaystyle \arccos x=\arcsin {\sqrt {1-x^{2}}},{\text{ if }}0\leq x\leq 1} arctan x = arcsin x x 2 + 1 {\displaystyle \arctan x=\arcsin {\frac {x}{\sqrt {x^{2}+1}}}} Whenever the square root of a complex number is used here, we choose the root with the positive real part (or positive imaginary part if the square was negative real).
From the half-angle formula tan θ 2 = sin θ 1 + cos θ {\displaystyle \tan {\frac {\theta }{2}}={\frac {\sin \theta }{1+\cos \theta }}}
arcsin x = 2 arctan x 1 + 1 − x 2 {\displaystyle \arcsin x=2\arctan {\frac {x}{1+{\sqrt {1-x^{2}}}}}} arccos x = 2 arctan 1 − x 2 1 + x , if − 1 < x ≤ + 1 {\displaystyle \arccos x=2\arctan {\frac {\sqrt {1-x^{2}}}{1+x}},{\text{ if }}-1<x\leq +1} arctan x = 2 arctan x 1 + 1 + x 2 {\displaystyle \arctan x=2\arctan {\frac {x}{1+{\sqrt {1+x^{2}}}}}}
sin ( arccos x ) = cos ( arcsin x ) = 1 − x 2 {\displaystyle \sin(\arccos x)=\cos(\arcsin x)={\sqrt {1-x^{2}}}} sin ( arctan x ) = x 1 + x 2 {\displaystyle \sin(\arctan x)={\frac {x}{\sqrt {1+x^{2}}}}} cos ( arctan x ) = 1 1 + x 2 {\displaystyle \cos(\arctan x)={\frac {1}{\sqrt {1+x^{2}}}}} tan ( arcsin x ) = x 1 − x 2 {\displaystyle \tan(\arcsin x)={\frac {x}{\sqrt {1-x^{2}}}}} tan ( arccos x ) = 1 − x 2 x {\displaystyle \tan(\arccos x)={\frac {\sqrt {1-x^{2}}}{x}}}
निम्नलिखित में k कोई पूर्णांक है।
sin ( y ) = x ⇔ y = arcsin ( x ) + 2 k π or y = π − arcsin ( x ) + 2 k π {\displaystyle \sin(y)=x\ \Leftrightarrow \ y=\arcsin(x)+2k\pi {\text{ or }}y=\pi -\arcsin(x)+2k\pi } cos ( y ) = x ⇔ y = arccos ( x ) + 2 k π or y = 2 π − arccos ( x ) + 2 k π {\displaystyle \cos(y)=x\ \Leftrightarrow \ y=\arccos(x)+2k\pi {\text{ or }}y=2\pi -\arccos(x)+2k\pi } tan ( y ) = x ⇔ y = arctan ( x ) + k π {\displaystyle \tan(y)=x\ \Leftrightarrow \ y=\arctan(x)+k\pi } cot ( y ) = x ⇔ y = arccot ( x ) + k π {\displaystyle \cot(y)=x\ \Leftrightarrow \ y=\operatorname {arccot}(x)+k\pi } sec ( y ) = x ⇔ y = arcsec ( x ) + 2 k π or y = 2 π − arcsec ( x ) + 2 k π {\displaystyle \sec(y)=x\ \Leftrightarrow \ y=\operatorname {arcsec}(x)+2k\pi {\text{ or }}y=2\pi -\operatorname {arcsec}(x)+2k\pi } csc ( y ) = x ⇔ y = arccsc ( x ) + 2 k π or y = π − arccsc ( x ) + 2 k π {\displaystyle \csc(y)=x\ \Leftrightarrow \ y=\operatorname {arccsc}(x)+2k\pi {\text{ or }}y=\pi -\operatorname {arccsc}(x)+2k\pi }